This project provided a better understanding of the intriguing navigational abilities of desert ants. Like many central-place foraging species, these animals return to their nests on a fairly straight path, even if the outbound foraging run has been very tortuous. I conducted several experiments in the Tunisian desert in close cooperation with Professor Rüdiger Wehner (Universität Zürich). These experiments focused on the accuracy and precision of the path integrator, its interaction with visual information such as landmarks (if available), and the systematic search strategies that these ants use as the final backup mechanism to find the nest.

a) Accuracy and Precision of the Path Integrator

Desert ants use path integration not only to return to the nest, a tiny hole in the desert ground (see left-most image below), as efficiently as possible but also to return to valuable food sources. Therefore, it is possible to establish feeders and to train the animals to forage to and fro between their nest and this feeder (see middle image below, showing a colour-marked Cataglyphis fortis that is leaving the feeder with a biscuit crumb). Colour-marking the ants enables an experimenter to compare the successive foraging trips of individual ants. Training ants to feeders allows for testing the accuracy of the path integrator of groups of ants that differ in their foraging routines, e.g. after they have been trained to feeders that were at different positions. By painting a regular grid of white lines on the desert ground (see right-most image below), I was able to record the ants' trajectories on graph paper, reduced in scale 100:1. Paths were digitised and transformed into cartesian coordinates for analysis.

     

Using these techniques, we could prove the long held assumption that the path integrator accumulates errors during foraging: the longer the foraging trip has been, the larger the overall error in the path integrator. The experiments also revealed that repeated training does not improve the path integrator in desert ants as even after an ant had visited the same feeder many times, the accuracy of its outbound and inbound runs did not improve significantly. Apparently, a higher accuracy from the beginning is ecologically more sensible than fine-tuning of the system during consecutive runs. In the ants' natural environment, food sources may vanish quickly anyway.

b) Interaction of Path Integrator and Landmark Information

Path integration is not the only means of navigation desert ants have at their disposal. However, due to their habitat which consists mainly of bare desert ground without any vegetation, most of the time they have to rely exclusively on their path integrator. Whenever available, however, they make use of visual information. I was the first researcher to show how the path integrator and landmark information interact during foraging excursions. Like in my other experiments (see above), I trained ants to artificial feeders and painted white grid lines on the desert ground in order to record the ants' paths. Some groups of ants were trained on the open ground, others had parts of their outbound and inbound runs flanked by black cylinder landmarks (see image on the right). The results of those experiments showed that landmarks have a remarkable impact on the foraging motivation of desert ant foragers. If the information provided by the landmarks and the path integrator are set into conflict, the ants tend to rely more on the visual landmark information than the directional information as given by the path integrator ("home vector").

c) Systematic Search in Desert Ants

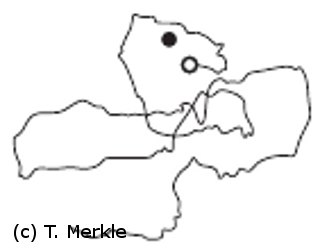
If an ant fails to find the nest after following the home vector it starts a systematic search for the nest. During this search C. fortis performs loops of increasing radius around the supposed nest position. It regularly returns to a region that is close to the starting point of the systematic search (open circle in left figure), i.e. the nest position as calculated by the path integrator, and then changes the direction in which it heads off next until it eventually finds the correct nest position (filled circle).

I compared the search patterns of groups of ants that differed regarding their foraging excursions and showed that the ants adapt their systematic search patterns to the uncertainty of the path integrator. That is, the longer the foraging excursion - and the larger the error accumulated in the path integrator - the wider the search loops. In other words, the ants account for this uncertainty by adjusting their search patterns accordingly. An additional experiment revealed that the searching behaviour is also influenced by the particular experience of the respective ant such as having foraged on familiar terrain. In summary, these experiments brought evidence that the systematic search in Cataglyphis fortis is not a fixed routine but a very flexible and adaptive behaviour.

Not only Cataglyphis ants, but also other desert arthropods (e.g. the beetle Parastizopus armaticeps and the woodlouse Hemilepistus reaumuri) return to their nest on a straight path even after a long and tortuous foraging excursion. This ability is based on their internal "path integrator", i.e. the integration of walking speed and angular variation along the walking route during foraging.

This mechanism provides the animal with the distance and direction of the nest from its actual position at any time, the so called "home vector". Reeling off this home vector allows the arthropod to return to the nest on a straight path. Nevertheless, after bee-lining back, the arthropod only rarely hits the entrance of its nest spot on. In this case, it starts a systematic search in the vicinity of its supposed nest, while extending the search radius more and more.

Together with Professor Wolfgang Alt and Dr Martin Rost, I developed a model that can perform the process of mathematically correct path integration. This model uses a combination of two coupled differential equations to calculate the arthropod's distance from the nest in cartesian coordinates. The model is egocentric, i.e. it has no fixed point of origin but the point of origin is the moving arthropod itself (see image on the left). This is biologically plausible as both sensory input and motor output signals are physiologically coupled. Therefore, it is naturally related to its two symmetry axes, the posterior-anterior axis and the perpendicular right-left axis. Thus, when identifying these symmetry axes with the X and Y axis of a cartesian coordinate frame (X,Y) and taking the arthropod's body centre as the origin (0,0), this constitutes a proper planar moving coordinate frame for representing the relative position of any object in the planar neighbourhood of the arthropod, e.g. its nest. The image shows the theoretical scheme of egocentric path integration by means of cartesian coordinates X,Y, specifying the position of the nest relative to the arthropod's body axes and determing the home vector G = (X,Y), here with X < 0 and Y > 0.

Given the arthropod's forward speed v and angular turning rate omega, one yields the following model equations for a precise update of the home vector (X,Y) during motion:

                                    

Our two-dimensional differential equation system is remarkably simple: it is linear in the two variable quantities X and Y, and it just uses the two speed input parameters as additive or multiplicative terms, v representing the rate of shift of the X coordinate backwards, and omega the rate of rotating the (X,Y) frame clockwise.

The above animation illustrates the model output for a natural outbound path (red) and the successive successful return path back to the nest (blue) based on precise path integration. Top: Plot of the paths in cartesian (x,y)-coordinates. Bottom: The values of X and Y over time as computed following the two differential equations (see above) provide sufficient information for the animal to return to the nest on a straight path.

For more details on our egocentric path integration model, please see our paper in the Journal of Theoretical Biology 2006.

Dr Robert Vickerstaff and I are currently working on a model of the systematic search behaviour of desert ants. This behaviour enables a forager to find the nest quickly even if the path integrator has failed to provide the exact position of the nest.

Habituation is a widespread form of behavioural plasticity in animals. It describes a decrease in responsiveness to events that are encountered frequently and repeatedly. The adaptive value in this decrease is the avoidance of unnecessary costs. However, since an error during the habituation process might be deadly, it is crucial that animals are very selective when they habituate. Therefore, it is likely that habituation is both context- and stimulus-dependent, thus involving some form of associative learning. However, only little is known about how changes in context or stimulus affect the habituation process, especially under natural conditions.

I investigated the rules of habitation in fiddler crabs (Uca spec.) in the context of predator avoidance. Fiddler crabs offer a good opportunity to study the ecology of habituation since large numbers of individual crabs, as well as all stimuli (natural and artificial) and contextual cues can be monitored and manipulated over extended periods of time. In addition, I could determine exactly what information the crabs had available based on their well studied visual abilities.

This project provided answers to some fundamental issues regarding the adaptive significance of habituation that had previously been neglected, despite being crucial for the understanding of the evolution of this form of behavioural plasticity. My results therefore advanced our understanding of how behavioural adaptations evolve under natural conditions and how sensory limitations constrain and shape animal behaviour.

The images above show the design and equipment used in my experiments with fiddler crabs. On the left: schematic aerial view of the experimental setup (not to scale); from his remote position, the experimenter moved a "dummy predator" (= a black styrofoam ball) by handling a fishing line that was stretched between two poles. On the right: Two video cameras fixed to metal poles 1.6 – 1.8m above the ground continuously recorded the crabs’ behaviour and their burrow entrances in the mudflat.

Fiddler crabs very strongly habituate to a close-by dummy predator when a short inter-stimulus interval is chosen as demonstrated in the following experiment:

The dummy was pulled from its starting position to its turning position, thereby moving 1m along the fishing line above the crabs, and immediately returned to the starting position (= one cycle). The average speed of this dummy movement was 0.2m/s, i.e. one complete cycle took approximately 10 seconds. Cycles were separated by 10 second intervals (= break). This exact experimental procedure of equi-duration cycles and breaks was repeated 120 or 180 times (i.e. for 40 or 60 minutes) before the first longer break occurred. The present analysis is focused on the first 50 dummy runs only. One should note, however, that experimental sessions with one setup in one location were continued to last between 70 and 100 minutes with varying cycle – break timings.

Figure above: Fiddler crabs strongly habituate to an aerial dummy predator when a short inter-stimulus interval is chosen. a,b) Percentages of active crabs are shown for the starting (blue line), turning (red line), and end point (black line) of 50 dummy runs (1 = first dummy run) with “active” defined as a) at two body lengths minimum from its burrow or b) generally visible in the recording area. For control purposes, the percentage of active crabs at time points 70, 50, 30, and 10 seconds before the first dummy run were assessed (x-values < 1). c) Sample size of analysed crabs for each dummy run. Crabs that were engaged in social interactions were excluded from the analysis.

Figure above: Crabs that did not descend into their burrows in response to the dummy became as explorative as before dummy run onset. Top) Percentages of active crabs that had reached distances of at least two body lengths from their burrows (> 2BL) at starting (blue), turning (red), and end point (black) of the dummy run. The pronounced decrease in activity during the first four dummy runs fully recovers to pre-dummy levels. Bottom) Sample size of analysable crabs for starting, turning, and end point of the dummy runs, i.e. crabs that did not remain underground.

Figure above: Differences in number of crabs active at the starting and at the end point of the first 50 dummy runs. Crab activity was defined as either a) a distance of at least two body lengths from the burrow or b) visible on the mudflat. Only the first exposure to the dummy triggers a change in activity while crab activity was not timed to the stages of each dummy movement in the consecutive dummy runs. Negative sign indicates that more crabs were active at the end than at the starting point of a dummy run.

Figure above: Mean distances between individual crabs and their respective burrows increase over time of dummy exposure. Top) Crabs ran to their burrows in response to the first dummy movement but started venturing out again during the consecutive four dummy runs. By dummy run number 23, crab – burrow distances reached previous levels at the starting (blue), turning (red), and end point (black) of each cycle for all crabs that were visible at the surface within the cameras’ field of view. Bottom) Sample size of crabs that remained exposed to the moving dummy.

During the course of this project, I was supported by grants from the German Research Foundation (DFG), the Centre for Visual Sciences (ANU), and the ARC Centre of Excellence in Vision Science (ACEVS).